Key 1 to Key 2 is a half step since they are next to each other.




A half step is not always from a white key to a black key.




In this example, Key 1 and Key 2 are still next to each other.




A whole step (or ''whole tone'' or simply ''tone'') is the same distance as two half steps.




Key 1 to Key 3 is a whole step.
Key 1 to Key 2 is the first half step. Key 2 to Key 3 is the second half step.




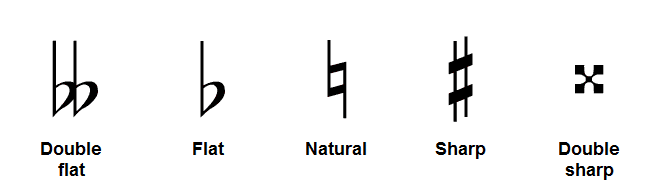
An accidental is a sign used to raise or lower the pitch of a note.




The first accidentals that we will discuss are the flat and the sharp.




The flat lowers a note by a half step while the sharp raises a note by a half step.




When typing, you can use a # to represent a sharp and a b to represent a flat.




Let's examine the black key in between C and D.




This key could be called C# since it is a half step above C.




It could also be called Db since it is a half step below D.




Another example would be E and F.




E could also be called Fb since it is half step below F.




Likewise, F could be called E#.




Whenever a certain pitch has multiple names, it is called an enharmonic spelling.




Next, let's discuss the double flat and the double sharp.




While flats and sharps alter a note by a half step, the double flat and double sharp alter a note by a whole step.




When typing, you can use a x to represent a double sharp and a bb to represent a double flat.




For example, both D and Ebb have the same pitch since you can reach D by going a whole step (or two half steps) down from E.




D also sounds the same as Cx since it is a whole step above C.




Finally, a natural cancels out any accidental and returns a note to its original white key.




We will learn more about naturals in an upcoming lesson.